Spatial Reasoning

An image of a building's geometric shapesSpatial reasoning—the ability to visualize, manipulate, and understand relationships between objects in space—is a critical cognitive skill. In this Spotlight, the first featured project enhances spatial visualization through dynamic digital representations of 3D geometry; the second develops early assessments to identify and support students’ spatial and numerical reasoning; and the third focuses on helping students navigate coordinate systems to improve graphing skills (which rely on spatial reasoning). Together, these efforts advance our understanding of how to support spatial reasoning development, which is essential for success in STEM education, careers such as engineering and healthcare, and everyday problem-solving. Read more about the featured projects and explore additional resources.
 
In this Spotlight:

Featured Projects

CAREER: A Transformative Approach for Teaching and Learning Geometry by Representing and Interacting with Three-Dimensional Figures Logo

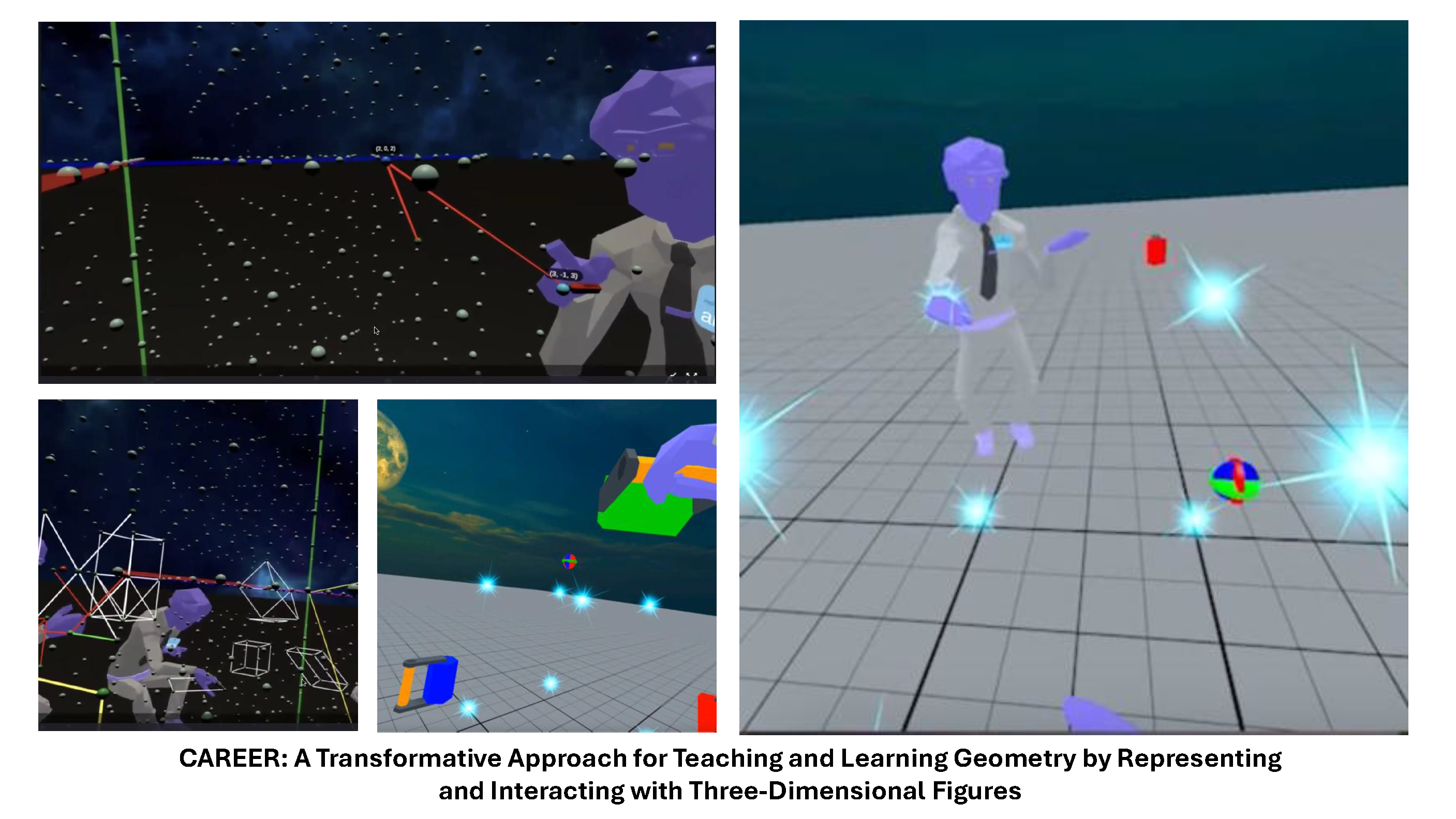
CAREER: A Transformative Approach for Teaching and Learning Geometry by Representing and Interacting with Three-Dimensional Figures

PI: Justin Dimmel
Grades: 9–11
Disciplines: This project examines the learning and teaching of geometry.

Project Description: This project is investigating how emerging technologies for representing and interacting with information in space— such as virtual or augmented reality— could create new opportunities for the learning and teaching of geometry. Reasoning about space is fundamental to tasks ranging from engine repair and moving furniture to multivariable calculus, linear algebra, and other advanced areas of mathematics. In the physical world, spatial reasoning is linked to physical movement, such as turning one’s head to get a different view of an assembly or rotating an object in space to fit it around a corner. Yet in the context of school mathematics, spatial figures are represented by two-dimensional diagrams that cannot be explored directly—a cube is represented as an overlapping network of unequal line segments and its interior is inaccessible. To bridge this disconnect, I am designing and developing geometry-specific, immersive virtual environments that allow groups of people to collaboratively explore three-dimensional figures that are represented as spatially inscribed, interactable diagrams. I am working with high school geometry teachers, as participant co-designers, to refine these immersive virtual environments and develop classroom activities they can do with their students. I work with the teachers to test the classroom activities with their students during special replacement lessons; for these lessons, my research team brings a set of head-mounted virtual reality displays to the school, and they video, audio, and screen record the classroom activity. The overall goal of this project is to describe the opportunities, and challenges, of teaching and learning geometry with interactive, digitally rendered, immersive, three-dimensional diagrams.

Innovative Technologies: The project is leveraging portable, lightweight, head-mounted virtual reality displays, such as the Meta Quest 3, to host immersive experiences where teachers and students can collaboratively explore a virtual world. The underlying hypothesis is that such worlds will help students develop their spatial reasoning skills by making mathematical figures accessible via the natural, movement-based routines we have developed to apprehend and navigate space.

Challenges and Strategies for Addressing Them: The current generation of consumer virtual reality head-mounted displays is relatively easy to use; still, it is somewhat of a production to bring virtual reality hardware into schools to study how teachers might make the most of these technologies to teach geometry. Generally, this is a multi-week process that involves (1) a visit to the the school in advance to test the compatibility of the hardware with the school's network; (2) several meetings with the teacher to plan the activities they will do in the environment; and (3) several hours of on-site prep time the day of the classroom visit to get the collection of 12 headsets online and set up the space where the activity will take place. Even with all of this planning, it generally takes 20 minutes of class time to get everyone situated in a virtual reality headset, and it might be 30 minutes before the teacher and students are ready to get into the heart of the planned mathematical activities. This is gradually getting better as these technologies become more common. One strategy we have identified is to refine the user interactions within in the virtual environments we have developed to make it easier for new users to learn the proverbial ropes.

Theoretical Frameworks: My project draws on work from embodied and enactive cognition; I am particularly interested in the potential for immersive, interactive, three-dimensional diagrams to create opportunities for students to learn about mathematics through coordinated movement.

Teacher and two students sit around a table in a classroom

Measuring Early Mathematical Reasoning Skills: Developing Tests of Numeric Relational Reasoning and Spatial Reasoning

PI: Leanne Ketterlin Geller | Co-PI: Lindsey Perry
Grade: K–2
Disciplines: This project targets mathematics through spatial reasoning, an area linked to success in career fields like engineering, computer science, and architecture. 

Project Description: The Measuring Early Mathematical Reasoning Skills (MMaRS) project is focused on developing high-quality, instructionally relevant assessments for kindergarten through grade 2 students that target two highly predictive early mathematics constructs: numeric relational reasoning and spatial reasoning. Spatial reasoning—how students mentally visualize and manipulate shapes, objects, and spatial relationships—is critical for STEM achievement; however, it is often under-assessed and under-taught in early education.

To support the instructional relevance of the MMaRS assessments, we grounded the content in the science of how people learn. We articulated learning progressions that detail how spatial reasoning develops in young children over time. These progressions were informed by thoroughly reviewing the existing literature, expert input, classroom teacher surveys, and cognitive interviews with students to ensure they accurately reflect how children think and learn.

With these learning progressions, we designed item models to assess students’ spatial reasoning. Items were tested using think-aloud interviews with students in kindergarten through grade 2 to evaluate the alignment between the intended and elicited knowledge and skills. Items were also reviewed by nationally recognized experts in early mathematics. These data were systematically analyzed and used to make final revisions to the items. Through this process, we developed instructionally relevant assessments of spatial reasoning skills for kindergarten through grade 2 classrooms.

Challenges and Strategies for Addressing Them: One of the unique challenges of our work is the necessity to provide K-2 teachers with resources that provide them with information so they can make better instructional decisions to support student growth. To address this, we utilized human-centered design principles to engage teachers in the design of our resources and assessment platform. By engaging educators in the co-design process, we ensure that our tools address the needs of teachers and can support decision making in meaningful ways.

What STEM Education Researchers Should Know About Spatial Reasoning: Spatial reasoning is foundational to STEM learning and careers, yet it is often narrowly defined in early education. Spatial reasoning goes beyond defining and classifying shapes; it includes skills like perspective taking, mental rotation, spatial visualization, and transformation. Even in early education contexts, students can meaningfully engage with various spatial reasoning tasks, such as mentally manipulate objects. By integrating spatial reasoning in early education, teachers provide students with opportunities to develop essential STEM-related competencies.

Products: Project Website

Suggested Reading: 

  • Ketterlin-Geller, L. R., McMurrer, J., & Barton, T. (2024). Designing classroom assessments with the end user in mind. International Journal of Testing, 24(2), 103–129. https://doi.org/10.1080/15305058.2024.2306549
  • Perry, L., Kuehnert, E., & Ketterlin-Geller, L. R. (2022). Promoting spatial orientation: Math activities for school and home learning. Teaching Young Children, 15(3), 8–12.
  • Pinilla, R. K., Wellberg, S., Castro, M., & Ketterlin-Geller, L. R. (2025). Analyzing children's spatial reasoning using an existing learning progression: Insights from interviews and task performance. Early Childhood Education Journal. https://doi.org/10.1007/s10643-025-01862-6

MSGGU LogoMiddle School Students Graphing from the Ground Up (Collaborative Research)

PIs: Hwa Young Lee, Teo Paoletti | Co-PI: Hamilton Hardison
Grades: 5–8
Disciplines: Though our project focuses on mathematics, graphical representations are ubiquitous across all STEM fields and are also utilized in non-STEM disciplines, such as social studies.

Project Description: We work with middle school students in small groups, and we conduct interviews in which we both examine how they make sense of graphs and support them as they develop their ideas about graphs. These teaching interviews address a variety of graphs, and we are especially interested in graphs that involve organizing space, like a map. We carefully observe how students create or interpret graphs to characterize their reasoning and to devise ways to support further development of such reasoning. For example, we ask students to describe the location of a buried treasure on a map, and we examine the frames of reference they use when describing locations in this spatial situation. We believe that identifying ways students make sense of spatial situations through graphs will help improve STEM teaching and learning by providing information that educators and researchers can use to better anticipate and build upon students’ available mental resources.

Instructional or Curricular Approaches: We have been designing tasks in the Desmos Activity Builder that teachers can use to support their students in constructing different types of graphs, including graphs that support spatial reasoning. These include activities in which students have opportunities to describe the locations of points in space for someone else or interpret descriptions of points in space to locate objects.

Innovative Technologies: We leveraged Desmos Activity Builder software to support the ways students organize space via dynamic and interactive visual representations that are not possible with paper and pencil. For example, we provided students with the option to layer various overlays of potential reference frames (e.g., vertical lines, horizontal lines, concentric circles) students could utilize in a spatial situation (e.g., https://tinyurl.com/desmosOverlays). These one-click buttons made it easy for students to efficiently access potential resources that they could draw upon when organizing spatial situations. We also used the Polygraph feature in Desmos to build a game similar to Guess Who in which students work together to find buried treasures by writing and interpreting descriptions about the treasure’s location, which promotes developing spatial reasoning and communication. (https://tinyurl.com/desmosBuriedTreasure).

Theoretical Frameworks: Our overarching goals are to advance knowledge of middle school students’ developing understandings of graphs “from the ground up” through attention to orienting features of graphs: coordinate systems and the reference frames that comprise them.

Our theoretical background focuses on students’ reasoning with reference frames—mental structures through which an individual gauges the extents of various attributes of objects or phenomenon (Lee, 2017; Levinson, 2003). Reference frames relate directly to the reference points and organizational structure (e.g., axes) an individual selects for, or imputes to, a coordinate system. By coordinate system, we mean a representational space in which an individual systematically coordinates quantities (Thompson, 2011) to organize some phenomenon. Typically, this space involves coordinating two (or more) frames of reference. For example, a Cartesian plane consists of two orthogonal axes on which frames of reference are overlaid so that the quantity on one axis can be coordinated with the quantity on the other axis.

What STEM Education Researchers Should Know About Spatial Reasoning: Oftentimes the reference frames and coordinate systems students are reasoning with when constructing or interpreting a graph are taken for granted or assumed to be similar to research intentions. All graphing activity is grounded in the underlying coordinate system and reference frames students are constructing, so it is important that researchers pay careful attention to these structures. 

Initial Findings: Through the project, we have been generating insights into the ways students construct and interpret coordinate systems. These findings include a novel framework for analyzing students’ construction and interpretation of graphs (Lee et al., 2023), identifying different ways that students organize spatial situations when they construct graphs (e.g., Olshefke-Clark et al., 2024), and the ways graphing conventions influence students’ reasoning (Gaspard et al., 2024).

Products:

  • Project Website
  • Publications
    • Olshefke-Clark, A., Paoletti, T., Margolis, C., Lee, H. Y., Hardison, H. L., & Gantt, A. L. (2024). Students’ meanings for coordinate systems: Continuous and ordered-discrete reference frames. In Kosko, K. W., Caniglia, J., Courtney, S. A., Zolfaghari, M., & Morris, G. A., (Eds.) Proceedings of the forty-sixth annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education. Kent State University (pp. 475–484).
    • Gaspard, B. R., Lee, H. Y., Paoletti, T., Tarigan, S., & Ford, L. (2024). “It does show it both ways, though.”: Emma’s reasoning through graphing conventions. In Kosko, K. W., Caniglia, J., Courtney, S. A., Zolfaghari, M., & Morris, G. A., (Eds.) Proceedings of the forty-sixth annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education. Kent State University (pp. 666–676).
    • Zolt, H., Gaspard, B. R., Lee, H. Y., Paoletti, T., Hardison, H. L., Ford, L., Tarigan, S., & Bui, M. (2024). Modeling students’ strategies when creating a graph: A focus on reference frames and coordinate systems. In Kosko, K. W., Caniglia, J., Courtney, S. A., Zolfaghari, M., & Morris, G. A., (Eds.) Proceedings of the forty-sixth annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education. Kent State University (pp. 695–701).
    • Paoletti, T., Margolis, C., Gantt, A. L., Hardison, H. L., Lee, H. Y., & Olshefke-Clark, A. (2024). Supporting learning through interpreting others’ solutions from a radical constructivist perspective: A theoretical report. In Kosko, K. W., Caniglia, J., Courtney, S. A., Zolfaghari, M., & Morris, G. A., (Eds.) Proceedings of the forty-sixth annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education. Kent State University (pp. 1640–1645).
    • Olshefke, A., Paoletti, T., Margolis, C., Gantt, A., Lee, H. Y., & Hardison, H. L. (2024). Different ways students interpret axes on graphs. In Evans, T., Marmur, O., Hunter, J., Leach, G., & Jhagroo, J. (Eds.) (2024). Proceedings of the 47th Conference of the International Group for the Psychology of Mathematics Education (Vol. 1). Auckland, New Zealand: PME (pp. 198).
    • Gaspard, B. R., Lee, H. Y., Bui, M., Hardison, H., Paoletti, T., Ford, L., Zolt, H., & Tarigan, S. (2024). Students’ reasoning through graph conventions. In Evans, T., Marmur, O., Hunter, J., Leach, G., & Jhagroo, J. (Eds.) Proceedings of the 47th Conference of the International Group for the Psychology of Mathematics Education (Vol. 1). Auckland, New Zealand: PME (pp. 265).
    • Lee, H. Y., Paoletti, T., Zolt, H., Bui, M., Hardison, H. L., Gantt, A. L., & Gaspard, B. R. (2023). A framework for designing tasks from the ground up. In Lamberg, T., & Moss, D. (2023). Proceedings of the forty-fifth annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education (Vol. 1). University of Nevada, Reno (pp. 88–89).

Additional Projects

We invite you to explore a sample of the other recently awarded and active work that focuses on spatial reasoning in the DRK-12 portfolio.

Related Resources

Additional DRK-12 Publications in the CADRE Library

Related Spotlights

 

Year